Cantilever Beams are members that are supported from a single point only; typically with a Fixed Support. In order to ensure the structure is static, the support must be fixed; meaning it is able to support forces and moments in all directions. A cantilever beam is usually modeled like so:
Cantilever Beam Deflection
Cantilevers deflect more than most other types of beams since they are only supported from one end. This means there is less support for the load to be transferred to. Cantilever Beam deflection can be calculated in a few different ways, including using simplified cantilever beam equations or cantilever beam calculators and software (more information on both is below).
Cantilever Beam Stress
Cantilever Stress is calculated from the bending force and is dependant on the beam’s cross section. For instance, if a member is quite small, there is not the much cross-sectional area for the force to spread across, so the stress will be quite high. Cantilever beam stress can be calculated from either our tutorial on how to calculate beam stress or using SkyCiv Beam Software – which will show the stresses of your beam.
Cantilever Beam Calculator
Got a complex cantilever beam? SkyCiv’s free cantilever beam calculator allows you to model and analyze complex beams to calculate cantilever beam deflection plus more. The software is extremely easy to use and requires no installation or download. Add your member length, then apply a number of different point loads, distributed loads and moments to your cantilever beam to get your reaction forces, bending moment diagram, shear force diagram and deflection results.
Cantilever Beam Equations (Deflection)
Taken from our beam deflection equation page:
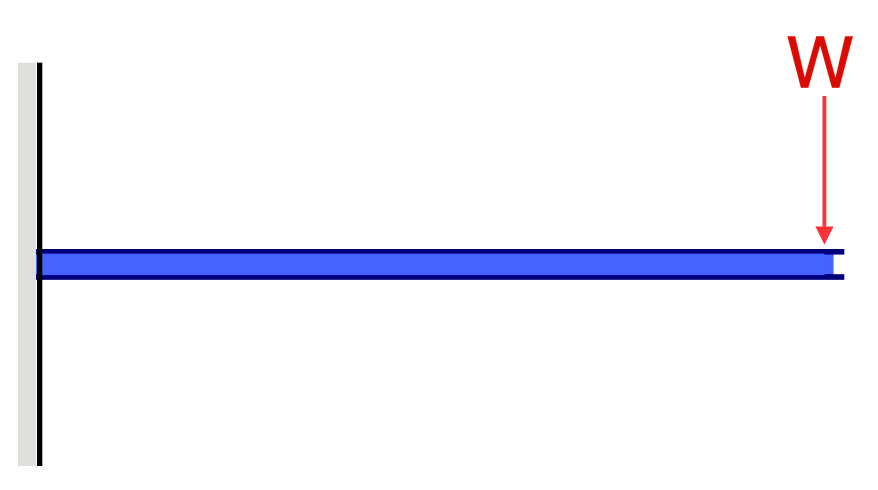
Sample Cantilever Beam equations can be calculated from the following formulae, where:
- W = Load
- L = Member Length
- E = Young’s Modulus
- I = the beam’s Moment of Inertia
 |
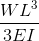 |
 |
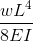 |
 |
 |
What is the maximum span of cantilever beams?
Usually, for small cantilever beams, the span is restricted to 2 m to 3 m. But the span can be increased either by increasing the depth or using a steel or pre-stressed structural unit. The span can be constructed long, given that the structure can counteract the moments generated by the cantilever and safely transfer it to the ground. A detailed analysis and design of the structure can help to study the possibility of long spanned cantilever beams.
How does a cantilever beam behave under loads?
A cantilever beam bends downwards when it is subjected to vertical loads. It can be subjected to point load, uniform load, or varying load.
Irrespective of the type of load, it bends downwards by creating a convexity upwards. This bending creates tension in the upper fiber and compression in the lower fibers. Hence, during the design of cantilever beams, the main reinforcement is provided to the upper fiber of the concrete beam, to withstand the tensile stress safely.
Source: skyciv